Logo de Sousa, J. D. (2002). Chess moves and their memomics: a framework for the evolutionary processes of chess openings.
Journal of Memetics - Evolutionary Models of Information Transmission, 6.
http://cfpm.org/jom-emit/2002/vol6/de_sousa_jd.html

Chess moves and their memomics: a framework for the evolutionary processes of chess openings

João Dinis de Sousa
Chess player with international rating by FIDE
Rua Actor Vale 49 4º Esq
1900-024 Lisboa  Portugal
j.d.sousa@oninet.pt, j.d.sousa@netc.pt

Abstract
1. Introduction
           1.1. Fundamental ideas and main claims of this paper
           1.2. Memetic introduction to chess
2. The world of chess and chess literature
3. The importance of openings: carriers of long lasting move memes
4. The dynamics of chess meme propagation
           4.1. Move recipemes, selectemes, and the instantiation of move phemes
           4.2. How players and chess organisms interact to propagate move memes
5. A framework for the memomics of chess
           5.1. Chess openings hyper-memome, loci, allelic competition
           5.2. Frequencies of moves in the meme-pool and in tournaments
           5.3. Outbursts of rapid proliferation
           5.4. Niche occupation and frequency-dependent selection
6. Chess organisms: basic analogies with living organisms
7. Evolutionary processes of openings
           7.1. Intra-specific, intra-organismic arms races and a Red Queen pattern
           7.2. Bootstrap of chess quality: a truly Darwinian process?
           7.3. Inter-specific arms races
8. Conclusion
Appendix
Glossary
References

Abstract

A framework for the study of chess in memetic perspective is outlined. The moves comprising a game are its main instructive replicators, and the game itself is an interactor or organism. Opening moves are potentially long-lasting, so complex evolutionary processes arise involving them. Positions are conceived of as loci, in which allelic moves compete, and the whole theory of openings is regarded as a tree-shaped hyper-memome, of which the memomes of games are branches. Several evolutionary processes of openings and their variations are discussed: frequency-dependent mechanisms, psychological niche occupation, co-evolutionary arms races, and the onset of high quality structures through the Darwinian process.

1. Introduction

1.1. Fundamental ideas and main claims of this paper

Look into figure 1. It shows the chessboard position (notice the coordinates) after the White move 1.e4 (meaning this moving the pawn from e2 to e4) and the Black move 1...e6 (meaning this moving the pawn from e7 to e6). For a full explanation of notation of chess moves see Appendix.

Figure 1. Opening moves are memes that last for long. See text
These two moves have been played since centuries, and they correspond to transmittable ideas in chessplayers minds, so they are memes. Moreover, they are early moves in a game (they belong to the so-called opening), therefore determining the structure of it, and are potentially repeated in millions of similar games. Games are memorized by players as victories, draws or defeats, are often reproduced in the literature, and can be considered the main interactors or organisms of move memes (like musical works are organisms of musical memes - Jan 2000). As genes determine the structure of biological organisms, so moves determine the structure of chess organisms (games). Thus, moves are instructive replicators which encode the phenotypic characteristics of games. Games exist in populations (tournaments, for example) and have patterns of health, development, and an ecology (section 6).

Knowledge of chess theory is not necessary to understand this article. It is important to imagine how the set of all possible chess moves is organized like a tree, starting from the initial position (for example, in the initial position, White has several possible moves; after White makes its first move, Black has also several possible moves, and so on; with each new move, thus, the number of branches of the tree at that level multiplies manifold). The tree of all possible moves is really gigantic but the important thing is that there is, embedded in it, a much smaller sub-tree called theory of openings, comprising the moves that "make sense", are played more often by players in real games, and are recorded and evaluated in chess literature . As will be seen, the entire theory of openings can be considered a tree-shaped "hyper-memome" (see section 5.1), full of memetic loci, in which alternative moves compete (like genes in genetic loci).

From this conceptualization, I intend to demonstrate that selection at the memetic level of chess moves generates complex evolutionary processes in chess openings, including arms races (these evolutionary processes will be explored in section 7). Openings are always evolving, year after year, expanding the existing theory of openings, and their evolution causes the emergence of high quality structures in games (for example, good pawn structures, well-placed bishops, safe king fortresses, etc). It is my contention (section 7.2) that these high quality structures are built by the fully Darwinian process operating through selection at the memetic level.

So, this article explores a memetic ecosystem where, if we make proper parallels between chess and evolution / memetics, which are basically,

moves = instructive replicators, memes
games = organisms, interactors
theory of openings = tree-shaped memome, in which moves compete as alleles
...we find the full Darwinian process operating and building patterns of adaptive quality in chess games (although adaptations in chess are not as complex as the ones shown by living organisms).

1.2. Memetic introduction to chess

Memes are units of cultural transmission (Dawkins 1976). This paper will focus on a specific class of memes that populates the minds of players in the international chess community: the ideas related to the chess moves themselves. The more chess is practiced, the more thousands of games and their underlying positions and moves are memorised by the player (a position is the current situation of all pieces in the chessboard at a given time, whereas a move is the act of moving one piece from one square to another). Alternative moves compete in the minds of players. Chess moves influence the outcome of chess games, and these outcomes determine repetition of the same moves in future games by the same player (which helps the longevity of the underlying memes in the same brain), and by other players (which is, in itself, an instance of meme replication to other brains). So, behavioural chess moves can be usefully conceptualised as phemes. A pheme is "a single memetic interactive trait which is the expression through some behavioural regularity of a meme at the level of selection" (Wilkins 1998). A variety of memes, other than the ones associated with chess moves, influence the eagerness of chess players to play the game repeatedly, and tend to spread from one mind to another: aesthetical considerations ("chess is beautiful"), the idea of money prizes to be won at tournaments, etc. However, move-related memes and their phemes (moves) will have a special focus here because:
  1. A chess game is totally determined by the moves played in it (in the same manner as an organism is determined by its genes). Moves are, like genes, discrete, particulate entities, and instructive replicators (the recipe-cake analogy applies to the relationship between moves and games).
  2. There are important and well-kept historical records of the games played by masters, and hence their moves, which facilitates future empirical studies of the epidemiology of chess memes and phemes, and the ecology and evolution of their phenotypes.
  3. The very nature of chess moves facilitates their description by means of a simple notation system comprising a limited number of "letters", that is, the information content of a move meme is essentially digital. The meaning of a move meme is kept through time without change.
This last characteristic raises, a priori, the likelihood of finding complex patterns of adaptation in the phenotypes of chess memes for the following reason. A Darwinian process can only generate adaptively complex phenotypic structures from the gradual accumulation of small ameliorations, in pre-existing not-so-bad structures. This process is helped by the durability of these structures in evolutionary time, which in turn is assisted by the fidelity of copy of coding replicators. Fidelity of copy can only be maintained in the long-term if the replicators express themselves in a digital form (Dawkins 1986; 1995). It’s unclear whether memes express themselves in a digital form in the brain (Dennett 1995). But the meaning of a chess move can be expressed digitally, even if its underlying ideas in the brain are encoded in a non-digital form. This makes chess moves potentially long-lasting in the chess community, which helps the development of complex patterns of adaptation in their phenotypic structures (games, tournaments, theories, etc). In section 7.2, I argue that the Darwinian process "recursively bootstraps quality" (Calvin 1997) in the patterns of chess openings. As will be shown, the "quality" built by this process consists of structures of the pawns and pieces well adapted to the chess strife, and recognized by chess theory as such, likely to produce, in the game continuation, good and more or less even prospects for both players (as will be seen, this relative equilibrium is indispensable for evolutionary survival of the patterns, because if a given opening produces victory or crushing advantage to one side, it will be avoided in the future by the other side).

I will focus on a special subset of extended phenotypes (Dawkins 1978, 1982) of chess memes: chess games. They are composed of positions and moves, which exist as behavioural phemes and also as memes in player’s minds. Games are not the only extended phenotypic effects of chess memes, but they have a "life of their own", they have an internal structure and complexity, evoke aesthetic feelings, interact with other human emotions (to be dealt with below), and are remembered as victories, draws or defeats by the two players involved and the audience. Given their complexity and interactions with the environment, they deserve to be called organisms, like musical works (Jan 2000), and texts propagated in the Usenet (Best 1997). The definition of an interactor as "an entity that interacts as a cohesive whole with its environment in such a way that this interaction causes replication to be differential" (Hull 1988), also applies to them. Chess games exist at behavioural level, and at that level, are usefully regarded as interactors (organisms), but they also exist as memeplexes in the brain (sets of tightly associated memes – the moves and positions – that often replicate together; however not always a given game is memorised in its entirety by a player, of course; and, unlike moves and positions, games almost never repeat themselves unchanged in the future).

The structure of chess games is entirely determined by the chess moves (phemes of chess memes) they are composed of. It’s important to note that, due to the overwhelming combinatorial possibilities of chess, a given game comprising more than 30 moves or so, is not likely to be ever repeated. An opening move (usually the first 10 to 25 moves of a game), on the other hand, is likely to repeat itself in many games, played by the same or other players.

As will be seen, the analogies between the world of chess moves and games and the world of biological organisms are interesting (section 5 and section 6). Evolutionary processes in chess openings are real (section 7), and are accounted for by a meme selectionist approach.

2. The world of chess and chess literature


According to the FIDE – Fedération Internationale des Échecs, there are presently 30,000 chess players with international rating (FIDE 2001), the cream of chess players worldwide. Several million other players play in official national tournaments but don’t have international rating. Thousands of tournaments (with hundreds of games each) are played annually worldwide, and of these, circa 2,000 count for international rating (FIDE 2001). In a given year, a player’s rating fluctuates according to his/her performance (dependent on his/her results and on the rating of adversaries). Games above a certain standard are published regularly in periodicals like the classical Belgrade-originated Chess Informant (Sahovski Informator Beograd 1998), ChessBase Chess Informant (ChessBase GmbH 2001a), and New in Chess (Interchess BV 2001). Soon after their publication, theoretical novelties in the opening of these games become incorporated in the theory of openings. The theory of openings is to be found in several encyclopaedias, published by several firms, but they usually conform to an international standard format, called ECO – Encyclopaedia of Chess Openings standard. The most complete ECO is the one published by ChessBase (ChessBase GmbH 2001b). It presently contains the openings of 1.1 million games, of which 60,000 are of high standard and commented on by reputed specialists. Thus, ECOs, chess informant journals and other periodicals provide vast banks of chess memes in extra-somatic form (Ball 1984) that "channel" chess meme proliferation to other players' minds in the near future.

World championship matches, especially ones in which the world champion is being challenged, attract a lot of attention in specialised publications, on the Internet, and in the media generally, and give rise to massive memetic proliferation of many of the opening moves and positions played.

3. The importance of openings: carriers of long-lasting move memes


For those unfamiliar with chess, the opening is the first part of the game, in which most pieces are developed to occupy active places. Very often, the structure of the entire game is determined by the opening. After the opening comes the middle-game, the phase in which most complex attacks, sacrifices, and turnarounds occur and in which a game usually reaches a climax in complexity and danger for one or both sides. If the game reaches a stage at which there are few pieces left, the remaining part is called the ending. There is a complex theory of endings too, but it is not as extensive as the theory of openings. Examples of classical treatises describing general principles of chess, applicable to openings, middle-game, and endings are Capablanca (1952), Golombek (1954), Karpov (1988), Fine (1990), and de Firmian (1999).

There is no set demarcation between the opening and the middle-game. A fuzzy criterion for considering the opening terminated is when most pieces have left their initial position. Another, the one I apply here because of its relevance to memetics, is simply when the moves cease to be described in the theory of openings (recorded in the main ECOs).

During the opening, the objectives of both players are development (positioning) of pawns and pieces in such a way that they are both prepared to launch an offensive and occupy good defensive positions. The position of the own king should be well defended, to avoid attacks from the opponent that could forcefully win. The seeds of an attack against the enemy king should be sowed, if possible. The pawns should be played in ways as to not compromise the "health" of the so-called pawn structure (an unhealthy pawn structure might produce defeat). Pawns and pieces should prepare for both offensive and defensive operations in the middle game to come. Sometimes, even the ending is already being prepared during the opening. The details of all this are very complex and only the reading of many treatises of the game, along with years of practice, can familiarize a player with them. However, it is clear that the structures arising from the opening (for example, a pawn fortress) show the hallmark of adaptation - they serve purposes, either defensive or offensive like, say, a predator's claw, and they are inherently complex. Section 7.2. will explore in more detail such patterns of quality.

At master level, the opening of a game is of utmost importance. If a player manages, at the opening stage, to stun an opponent with a novelty or a move s/he doesn’t know, s/he will bring the opponent into his/her "theoretical territory", and probably gain an advantage that might lead to a rapid victory. Openings are very diverse, and a player cannot know everything, so s/he usually specializes in a repertoire of several openings when playing with White, and several others when playing with Black. Additionally, masters even take into consideration, prior to a given game, whom they will be playing against, and do specific homework for the event, in the hopes of producing a stunning effect. Electronic databases like ChessBase, which permit player-based searches, can be useful tools in such preparation.

The opening is the most relevant part of the game to memetics simply because opening moves are often repeated in many games and populate the minds of chess players, and the vast written theory of openings (a given 5th move in an opening is likely to be inserted as a meme in the minds of thousands of players at a given year, whereas a given 40th move was probably played only once in the entire history of chess).

Chess books often contain historical games with brilliant moves made in the middle-game (the so-called combinations, usually involving sacrifices of pieces and leading to a forceful win). For example, two games in the nineteenth century became known as "The Immortal Game" and "The Evergreen Game", respectively, due to the brilliant combinations involved in them (Horowitz 1961). Whilst combinations are also successful memes, and knowledge of them contributes to a player's expertise in the middle-game, they will not be emphasised in this article because they, unlike opening moves, cannot be repeated in new games, and so there are no evolutionary processes based on them. See figure 2 for one of the most fantastic combinations in the history of chess.

Figure 2. A brilliant combination (and prolific chess meme). White plays 1.Re7+ (the rook in e1 captures in e7), Black responds 1...Ne7 (knight in c6 captures that rook), 2.Qd7+ (White queen is sacrificed in d7!) 2...Kd7 3.Bf5+ Ke8 4.Bd7+ Kf8 5.Be7 mate (White wins). A rook and the queen are sacrificed to achieve mate. This game was played between A. Anderssen and J. Dufresne in Berlin, 1853, and became known as "The Evergreen Game" (Horowitz 1961). As this diagram was later reproduced in innumerable books, the combination is certainly a good meme. However, such memes are not the main subject of this article because they, unlike opening moves, are never repeated in real games in posterity, and so, they don't generate the evolutionary processes of openings which are our main interest here. Putting it simply, combinations don't evolve, openings do. The study of combinations is important, however, to improve a chessplayer's technique.

4. The dynamics of chess meme propagation

4.1. Move recipemes, selectemes, and the instantiation of move phemes

What ideas are associated with a chess opening move? Such ideas, which I will call COMRM - Chess Opening Move Related Memes, certainly include:

  • a simple representation of the move itself
  • a "mental picture" (even if incomplete) of the position in which the move is to be played
  • practical evaluations: "Is it good?"; "Do I often win with it?"; "Did Kasparov win with it last year?"; "Is it favoured by experts in the ECO?"; "Is it consonant with my style?"
    • In addition to these, other ideas are evoked: memories of past games in which the player played the move, memories of famous games taken from the literature that included the move, aesthetical feelings about the move, etc, but these are not clearly memes (they don't often propagate to other brains, and are memories specific to just one person). They can be called mnemons (Lynch 1998). Types a, b and c are clearly memes. A move meme is more likely to be instantiated in real behavioural moves made in real games, if it is known by the player (types a and b exist in his/her brain), and if the practical evaluations described in c are positive. However, this is just a very rough psychological model of chess move memes. This article doesn’t aim to explore the psychological aspects, but rather the memetic evolutionary processes. Memes of types a and b are recipemes (competing ideas of how to do things - Langrish 1999), while memes of type c are selectemes (ideas about the value of other ideas - Langrish 1999), or meta-memes (Hales 1998).

    The behavioural instantiation of an opening move meme in a real game is a move pheme. If a player knows and likes an opening move s/he is more likely to play it, but his/her play will also depend on the opponents. So, for example, if a player knows and likes a move, the associated COMRM are stored (enduringly? permanently?) in his/her mind, and s/he may instantiate the behavioural move pheme 10 times a year in official tournaments and 100 times a year in friendly "blitz" games (unofficial games played with very limited reflection time). These behavioural moves and the resultant games and outcomes will update the evaluative meta-memes in the mind of the player, influencing his/her later repetition of moves, and propagation to other players' minds via direct observation, personal communication and literature.

    4.2. How players and chess organisms interact to propagate move memes

    Figure 3 shows the dynamics of chess meme transmission. COMRMs often instantiate themselves in move phemes. The latter form chess games, which are reproduced (when the standard of play is high) in chess periodicals, in which moves, positions and games receive expert commentary (often by one of the players - usually the winner). If the game has some theoretical novelty, its opening will be included in subsequent issues of ECOs. Expert teams comment on, classify, order hierarchically and evaluate relevant moves and positions. Commentaries, classifications and evaluations, together with the games themselves, determine propagation of the underlying memes to the chess community.


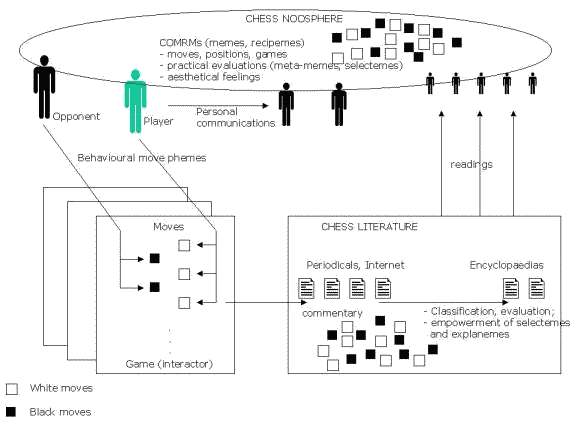
    Figure 3. Dynamics of chess meme propagation; see text, sections 4.1 and 4.2

    5. A framework for the memomics of chess

    5.1. Chess openings hyper-memome, loci, allelic competition

    From here on, I will first endeavour to build a conceptual framework that I believe will be useful to understand the evolutionary processes of chess openings and the ecology of chess games. My main aim is to draw comparisons between the world of chess and biology that might shed light on the evolutionary processes of chess openings.

    In the framework I propose, main replicators are opening moves. A game is mainly determined by its opening moves, that is, the latter comprise its memome (opening). The set of moves in a memome is small (usually 10 to 25 each side). The set of opening moves recorded in the theory of openings (written in the ECO, see section 2) is much bigger. At the start of a game, White may choose from several possible first moves. These moves may be called alleles, and there is obvious competition between them at the memetic level. Following White’s first move, Black has many alternative responses. These responses are, therefore, in allelic competition among themselves, but are neither in competition with White moves, nor with Black moves made in other positions. In other words, a given position, for operational purposes, is a locus, wherein the different possible moves compete.

    For example, White’s possible first moves 1.e4, 1.d4, and 1.c4 (see Appendix for the notation of chess moves) have been in allelic rivalry for centuries (Keene 1990).

    I propose to call to the entire recorded theory of openings COHM - chess openings hyper-memome (or hyper-memomic tree). My choice of the word "hyper-memome" instead of just "memome" simply aims to keep "memome" associated with a single organism (that is, chess games (organisms) have their own memome, or set of defining opening moves, just as living organisms have their genome). The chess hyper-memome is obviously organised like a tree (unlike the genomes of living organisms), with branches diverging from the initial position (first locus). A memome (the opening of a game) is a complete branch in the hyper-memomic tree. Figure 4 helps to see all this more clearly. The COHM is always evolving new branches, as the community of players invents theoretical novelties. A novelty creates both a new move (allele) in the position (locus) it belongs to, and a new position (locus), expanding, in this way, the COHM tree. This property of chess novelties has no parallel in the mutations of living organisms. For this reason, the chess memomics framework outlined here does not exactly parallel the genomics of living creatures, but I believe it is still illuminating in the sense it will clarify the ongoing evolutionary processes of openings.

    The COHM can be easily operationalised by considering it equivalent to the tree of openings depicted in the leading ECOs, that is, an ECO is the extra-somatic map of the hyper-memomic tree. In the minds of players, at best fragments of the COHM exist (even the most bookish of grandmasters cannot know everything about openings).

    Table 1 summarizes this framework, with classification of chess concepts in memetics terminology.
     

    CHESS ENTITIES MEMETICS TERMINOLOGY
    Ideas about chess opening moves memes, recipemes, selectemes (in allelic rivalry)
    behavioural opening moves phemes
    Total set of ideas about chess opening moves in the community of players meme-pool
    a position in an opening memetic locus
    the opening of a real game memome of an organism
    theory of openings (as recorded in the ECOs) COHM, hyper-memomic tree
    a game interactor, organism
    a tournament population of organisms
    a classified opening species
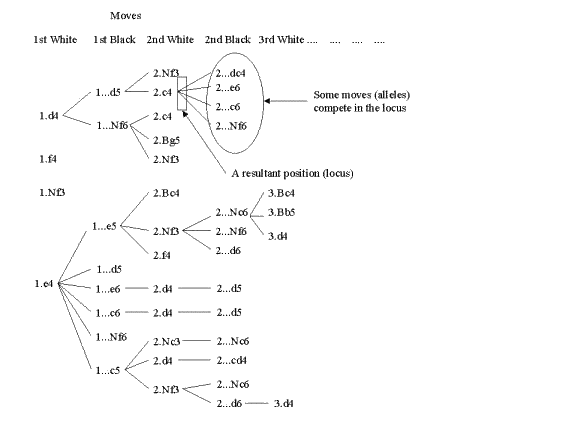
    Table 1. Chess concepts and their memetic classifications

    Figure 4. The beginning of the chess opening hyper-memomic tree, with many popular openings. The set is not exhaustive. As the diagram shows, each position is a locus in which several allelic moves compete.
    In the framework I have outlined, there is even a chess equivalent of a supergene - a set of genes at different loci (usually closely linked) that tend to appear together in the same individual (Maynard Smith 1998). For example, in the Sicilian opening (defined by 1.e4 c5) both sides usually play the following sequence: 3.d4 cd4 4.Nd4 Nf6 5.Nc3. Neither side normally deviates from these moves, so they propagate as if they were a "supermove".
     

    5.2. Frequencies of moves in meme-pool and in tournaments

    Empirical studies of chess memetics are likely to be more interesting if focused on the earliest phase of opening (the first 5 to 7 moves), because it is in this phase that known moves are more widely played, that is, their behavioural phemes achieve higher frequencies in the thousands of tournaments played in a given year. Frequencies achieved at the phemetic level might be very different from the frequencies of implied memes in the meme-pool (brains of players). For example, consider the alleles competing in the initial position. The most popular are 1.e4, 1.d4, 1.c4 and 1.Nf3 (ChessBase GmbH 2001a). At behavioural level, the frequencies of the associated phemes are complementary (their sum approaches 1). In the minds of players, the frequencies depend on whether one is speaking of the associated recipemes or selectemes (Langrish 1999). Recipemes about them all have a frequency close to 1 (everybody knows these initial moves), but the associated selectemes (favourite status among players) also have complementary frequencies (some players prefer and specialize in 1.e4, others in 1.d4, etc). At later stages of the opening, for example, at move 10 or so, there are many branches of the hyper-memomic tree, so fewer chess players know a given position and its alternative continuations, or, in other words, in these hyper-memomic regions, even recipemes have lower frequencies in both meme-pool and as behavioural move phemes. Move alleles compete at three different levels, as recipemes, as selectemes and as phemes.
     

    5.3. Outbursts of rapid proliferation


    Outbursts of rapid proliferation do occur. Here is a typical situation. In a given position, several move alleles exist and one of them is seldom played. Afterwards, an event attracts attention to that move: either new analysis favours the move or some important grandmasters play it in a handful of games. The media spread news of it and thousands of chess players repeat the move all around the world. An example of this happened with the move 6.Be2, after the opening sequence 1.e4 c5 2.Nf3 e6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 d6, in the late eighties, according to Karpov: "In recent years the bishop has usually been developed on e2 in the Scheveningen Variation, which was probably influenced by my first two matches with Kasparov" (Karpov 1988, p.21). In this phase, rapid proliferation at phemetic level is led by proliferation at recipeme level (more people know it). Later, more exhaustive analyses around the variations derived from the move, helped by the greater number of masterly games played with them, will reveal the value of the move relative to its alleles. If outcomes of games and analyses indicate that the move was not so good after all, its population at phemetic level starts to fade again. At this phase, phemetic decay is led mainly by selecteme change.
     

    5.4. Niche occupation and frequency-dependent selection


    Moves occupy ecological niches in their environment (minds of players). Some moves and resultant openings are preferred by enthusiasts of sharp positions and aggressive playing, others by enthusiasts of defensive positions and slow strategic manoeuvring. According to Fine (1956), there is a negative relationship between the personality of chess players and their style: aggressive people play quiet games and vice-versa. The relationship is probably not so simple, and other personality dimensions, such as a sensation-seeking tendency, also affect preference for sharp positions. Games derived from 1.e4 are generally sharper than the ones derived from 1.d4, and it's not obvious which one is more effective, so players' preferences for them derive mainly from their own styles (and possibly personalities).

    Frequency-dependent selection mechanisms clearly exist in chess. If a move becomes rarer than it had been previously, and providing it is not a decidedly bad move, the few players still playing it will gain an edge, because less opponents will be familiar with it. This mechanism probably keeps many moves alive, even when they have been labelled inferior by theorists. Authors often encourage players to include in their repertoire relatively neglected variations (Kotov 1982).

    6. Chess organisms: basic analogies with living organisms

    The following are analogies between chess games and living organisms. They help to enliven the discussion of evolutionary processes in section 7, and illustrate the usefulness of regarding chess games as organisms.
    a) Healthy  b) Healthy  c) Unhealthy
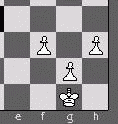
    d) Unhealthy e) Unhealthy f) Unhealthy


    6.1.1 A note on the validity of the chess organism concept

    Is it far-fetched to consider the end of a game (through victory of one side, for example) the "death" of it as an organism (after all, players are actively pursuing victory)? Aren't the players the main interactors or organisms of chess memes? Does the analogy between chess organisms and living creatures runs short by the fact that a chess game hosts an internal struggle with two sides trying to win?

    First, chess games could be always conceived as organisms in a broader sense, like musical works (Jan 2000) and messages propagated in Usenet (Best 1997), in the sense of structures that function as interactors of their underlying memes. In the present section, I contend something more specific: that there are interesting analogies between chess games and living organisms. I don't contend that chess games are really alive, of course, just that they have the analogies to living organisms described above in this section (reproduction, development, health, bauplans and senescence). As always with memetics, humans are by far the most important interactors of memes and this applies to chess too. Human chess players, authors and theorists are the main interactors of chess memes (as musicians and listeners are the main interactors of musical memes), but it is still useful to look for other interactors, and chess games show the important features already mentioned and besides they are fully prescribed by moves (like living organisms are prescribed by genes). As for chess games hosting an internal struggle between two sides, this is not unseen among living organisms: symbionts like mitochondria are often in conflict with the nuclear genes of their hosts, causing observable effects (Hurst et al. 1996), and an internal conflict exists between the limbic brain and the cortex of mammals in general, including humans, for the sake of evolutionary arms races between paternally and maternally imprinted genes (Keverne et al. 1996).

    7. Evolutionary processes of openings

    While the last section explored analogies between biological organisms and chess organisms, sections 7.1, 7.2, and 7.3 go a step further and explain evolutionary processes in chess openings led by memetic natural selection.
     

    7.1. Intra-specific, intra-organismic arms races and a Red Queen pattern

    Within openings, there are long-lasting arms races between the White and Black sides. Each side evolves novelties to gain an advantage over the other. Openings acquire new variations (branches in the hyper-memomic tree) over time in this process. With the new variations, new plans and defence and attack strategies emerge. In general, White has the upper hand, which it owes to its first move advantage, but Black has many defence and counter-attack opportunities. Arms races occur within a given opening (species), so they are intra-specific, and might be called asymmetrical (Dawkins & Krebs 1979), since they happen between White and Black sides, which develop different formations ("behaviour") and have opposing interests. These arms races are even intra-organismic, since two different sets of replicators in the same organism (White moves and Black moves) co-evolve antagonistically, as happens with different sets of genes in the same living organism (examples of intra-organismic genetic arms races involve cytoplasmic genes against nuclear genes (Hurst et al. 1996), and maternally imprinted genes against paternally imprinted genes (Moore & Haig 1991; Keverne et al. 1996)).

    ECOs can be used to analyze the evolution of a given opening, because they contain all variations composing it, and the references to the historical games played in each variation include the names of the players and year of play.

    Keene (1990) describes briefly the "pedigrees" of the following openings: King's Gambit (1.e4 e5 2.f4), Ruy Lopez (1.e4 e5 2.Nf3 Nc6 3.Bb5), Sicilian (1.e4 c5), Queen's Gambit (1.d4 d5 2.c4), French (1.e4 e6), Caro-Kann (1.e4 c6), English (1.c4), Reti (1.Nf3), King's Indian (1.d4 Nf6 2.c4 g6 3.Nc3 Bg7), and Nimzo-Indian (1.d4 Nf6 2.c4 e6 3.Nc3 Bb4). Some originated in the sixteenth century and have been evolving ever since. From the names of many of the openings, it is easy to tell that speciation occurred in an allopatric fashion (although allopatry of chess openings is not governed by the same logic as allopatry of biological organisms; there is not even a chess equivalent to a species originating from a previous one).

    In each opening, year after year, novelty moves expand the hyper-memomic tree, causing new positions and variations to appear in the theory of openings and to proliferate at the phemetic level (as games played in tournaments). This is, in itself, evolution of openings. One way to grasp the evolutionary trends of an opening is to compare the chapter of an ECO referring to that opening for two editions of the ECO compiled at different times. This comparison is done below for the Sveshnikov opening (determined by the opening moves 1.e4 c5 2.Nf3 Nc6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 e5). See figure 7 and table 2.

    Figure 7. The Sveshnikov opening (1.e4 c5 2.Nf3 Nc6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 e5). It is analysed in the chapter B33 of the ECO (Sahovski Informator Beograd, 1978, 2002).
    In 1978, this opening was seldom played by masters. Consequently, it showed few variations and analyses in the ECO compiled in that year (Sahovski Informator Beograd 1978). During the 80s and the 90s, it gained greater popularity, and this caused massive evolution of its variations, through the appearance of hundreds of novelties. Then, looking at a more recent issue of the ECO (Sahovski Informator Beograd 2002), it is apparent a great increase in number of variations and analyses. See table 2. This great increase is a mark of evolutionary success. It didn't happen to most openings, I selected one it happened to. The increase in number of variations analysed in the ECO is associated with an increase in number of games played annually with this opening (ecological success). Thus, evolutionary success of an opening is usually accompanied by ecological success of its games.
     
      ECO, 1978 issue ECO, 2002 issue
    Number of pages 6 25
    Number of primary variations 14 62
    Number of footnotes (with both secondary variations and analyses) 73 377
    Table 2. Statistics for the Sveshnikov opening, as it appears in the ECO, for the years 1978 and 2002. Notice the great increase in number of pages, primary variations and footnotes. Primary variations are variations more often played and resulting from selection among many secondary variations. Primary variations are deeply analysed by the ECO contributors (pre-eminent grandmasters), and represent usually what is best for both White and Black sides. Footnotes contain secondary variations (deviations from primary variations), analyses and evaluations (meta-memes in extra-somatic form). See Sahovski Informator Beograd (1978, 2002), chapter B33.
    Which novelty move memes are likely to expand the hyper-memomic tree of openings successfully, in such a manner that the newly explored positions and variations will proliferate in the meme pool and at the behavioural level, later on? Certainly not losing moves. And certainly not winning moves either. A winning move is a move that wins the game forcefully, regardless of the opponent’s subsequent continuation. Every player wants to play a winning move, but they also want to prevent their opponent from doing so. If, say, White’s 14th move is a winning move, then Black’s 13th move, played immediately beforehand, was a losing move, because it led to a losing position. Therefore, in future games, players who have understood what happened will avoid playing the same position with Black. As a result, both the Black losing move and White winning move become rarer, at the phemetic level, in subsequent tournaments (although many players may still be playing them if they are not aware of the situation). Therefore, variations that have been shown to lead to one side’s forceful victory, usually cease to be played at masterly level, and also cease to evolve further, though they may still continue to be described in the ECOs, correctly classified as hopeless.

    An example of this is given by a variation of the Sicilian Scheveningen opening, a particularly sharp one (see figure 8).

    Figure 8. A case of quasi-extinction. The sharp variation 1.e4 c5 2.Nf3 d6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 e6 6.g4 d5 leads to great White advantage. See text.


    The position seen in figure 8 belongs to a variation ranked as a primary variation in the ECO of 1978 (Sahovski Informator Beograd 1978). However, even at that time, the variation was classified in the ECO as "clear White advantage". In fact, later evolution of this variation brought new masterly games and analyses which proved that White had too good prospects. Then, in the 80s and 90s, the variation started to be avoided by Black, and the 2002 edition of the ECO (Sahovski Informator Beograd 2002) barely mentions it, classifying it as "clear White advantage". So, the variation almost ceased to be played (no one wants to play it with Black). This is an example of how, in the theory of openings, variations that give great advantage to one side bring evolutionary deadlocks.

    Therefore, evolutionarily successful novelty move memes are the ones that expand the tree with playable, interesting, resourceful, agreeable variations and positions for both sides, while maintaining some equilibrium. In fact, after centuries of evolution, and for each of the major openings, most widely played variations are evaluated in the ECOs (ECOs include such evaluations, made by master experts in each opening) as either equality (meaning that the game tends naturally to a draw, or at least, equal chances for both sides) or slight White advantage (meaning that the probability of draw is high, although White still has some chances of tipping the balance in its favour). Variations of slight White advantage are more common than variations of slight Black advantage, reflecting White's first move advantage sometimes lasting till the 25th move (ChessBase GmbH 2001b).

    There is an obvious analogy here with the Red Queen (Van Valen 1973). In the chess theory of openings, "everything is running to stay in the same place", in the sense that White and Black strategies, plans, and formations co-evolve antagonistically, without any of them getting the upper hand in the long run.

    An illustration of this Red Queen effect is shown by the evaluations of the Sveshnikov (the opening already discussed, which had outstanding evolutionary success in the 80s and 90s) primary variations made by ECO contributors. See table 3.
     

    Classification (meta-meme) of position (at the end of a primary variation) Meaning of the classification Number of primary variations with this classification (Sveshnikov opening, chapter B33, 2002 issue)
    Stable equality The position is even and stable 17
    Unstable equality The position is more or less even but not totally clear, and still has tension 12
    Compensation for material The position is even, the material advantage of one side is compensated by initiative of the other 11
    Slight White advantage White has a slight advantage, not enough to victory 20
    Slight Black advantage Black has a slight advantage, not enough to victory 0
    Clear White advantage White has a clear advantage, it may be enough to victory 2
    Clear Black advantage Black has a clear advantage, it may be enough to victory 0
    Decisive White advantage White wins forcefully 0
    Decisive Black advantage Black wins forcefully 0
    Table 3. The equilibrium of an evolutionarily successful opening. As shown, of the 62 primary variations of the Sveshnikov opening (chapter B33 of the ECO, Sahovski Informator Beograd 2002), the overwhelming majority leads to equilibrated or even positions (first 3 rows) or slight White advantage (4th row), after decades of evolution. No primary variation leads to decisive White (or Black) advantage. Then, in the White-Black arms race, after decades of evolution, no side got the upper hand. This equilibrium is characteristic of most openings.

    7.2. Bootstrap of chess quality: a truly Darwinian process?

    The outcomes of enduring chess arms races are opening variations and positions that go on satisfying players through time. Evolutionarily successful variations contain neither losing nor winning moves (see section 7.1), and provide acceptable resources for both sides: good pawn structures, good king fortresses, giving relative security to both kings, a more or less even control of the central squares, good locations for pieces, including the control of important diagonals by bishops and important columns by rooks, good (but not overwhelmingly advantageous) chances of attack, perspectives of acceptable endings... It is as if opening variations evolved "adaptations" similar to the ones shown by living creatures. See diagram in figure 9.

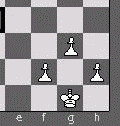
    Figure 9. Patterns of adaptation in openings. The position is reached after the opening sequence 1.e4 c5 2.Nf3 d6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 g6 6.Be3 Bg7 7.f3 0-0 8.Qd2 Nc6 9.Bc4 Bd7 10.0-0-0 Rc8 11.Bb3 Ne5 12.h4 h5 13.Bg5 Rc5, a variation in the Sicilian Dragon opening. See text.

    7.2.2 Patterns of adaptation (high quality structures) in the position of figure 9

    In this position, all bishops dominate important diagonals. The bishop in b3 exerts pressure on the f7 square, near the Black king. The bishop in g5 points to square h6, to which it may go later, to be exchanged by the powerful Black bishop in g7. Both sides have their knights centralised. White rooks are also well placed: one dominates the central column d, the other prepares an attack on the Black king through the h column (Karpov 1988 discusses these themes in this opening, along with analyses of many games with it). Both kings are massively defended by pawns and pieces. Black rook in the c column is already putting pressure on the White king fortress. The pawn structures are healthy and guarantee a later acceptable ending (if the game goes that far). Pawns in e4 and f3 restrain the mobility of the Black knight in f6. Both sides have good, but not yet decisive, chances of attack. Patterns of adaptation are apparent, in this, and in most other memetically successful openings. A survey of chess literature (for example, Capablanca 1952; Kotov 1982; Karpov 1988) shows that my description of the qualities of the position corresponds to evaluative criteria present in chess theory. In other words, these qualities are what chess players want.

    Although I refer to some chess formations as being adaptive, I'm conscious that their adaptiveness is not as complex and "fine-tuned" as the one displayed by living organisms (nor could it be, since the chess "world" is a board with 64 squares and up to 32 pieces inhabiting it, contrasting with the billions of molecules of a living organism). The expression adaptive complexity should not be used when referring to chess formations, because of the informational exiguity of a 64 squares board. But still chess formations are adaptive in the sense of being good for attack and defence, and providing players with good and resourceful continuations of the game, therefore enjoying the preference of players and appraisals in the literature.

    Are these marks of quality truly Darwinian, that is, do they stem from natural selection of chess memes in the meme-pool? Is it more accurate to regard these patterns as the product of direct human invention? Are they just the result of a "carving" selective process (Calvin 1997)?

    Players do invent the chess novelties, but this process is essentially different from the appearance of the adaptations I mentioned. When a master "discovers new territory" in the hyper-memomic tree, by creating a novelty, s/he is basically unaware of all evolutionary races that will follow in the subsequent variations derived from the novelty in years to come, and also largely unaware of the efficacy of his/her novelty. At best, s/he is able to figure out a good continuation during the game s/he is playing, and perhaps to win that game. His/her discovery may be likened to the work of an artist or scientist, the product of mental simulation of the game's continuation. But the proliferation of the shown position and its adaptive structures is an entirely different process, influenced by the outcomes of hundreds of future games derived from the new variation, and by the interplay of the associated recipemes and selectemes in the chess noosphere.

    To put it more clearly, let’s turn again to the position of figure 9. The position arises after a displayed sequence of 10 opening moves by each side. Each of the moves (say, the last Black move, 13...Rc5) must have come about as a novelty played by someone in the last centuries, but for each novelty, the player who invented it had no chance to foresee the evolutionary impact of the new move in the tree of openings. The shown opening "lives" in thousands of chess games played each year with it. And all openings of the theory of openings with a proliferation comparable to this are just a very tiny minority of all variations already played, let alone of all possible variations (the whole known theory of openings, or hyper-memomic tree, is a small tree in a gigantic, virtually infinite, space). For example, in the last 50 years, as recorded in ECO (Sahovski Informator Beograd 2002), White's 10th move (10.0-0-0) has had two main alleles (10.Bb3, 10.h4) but 10.0-0-0 became much more popular than the others; Black's 10...Rc8 has had four main alleles (10...Qa5, 10...Qb8, 10...Qc7, 10...Na5), and of these, only the first was played with a good frequency, but in the last 15 years or so, it has been overtaken by 10...Rc8 (Sahovski Informator Beograd 2002).

    The opening is prolific thanks to its adaptive qualities (described in the second paragraph of this section). These qualities guarantee that its many sub-variations (the many possible continuations after the 13th move, say, from the 14th to the 20th or so) lead, on average, to more or less even possibilities for Black and White (albeit with a slight White advantage), with good chances of attack and defence for both sides, with perspectives of acceptable endings, and generally satisfying the players.  As already seen, if the shown position was won to any side, or if the chances of one side overwhelmed the chances of the other, the variation would have lost its popularity long ago (it takes two people to play). Accordingly, and as with the Sveshnikov opening (see last section), a pattern of equilibrium can be seen in the meta-memetic evaluations recorded in the corresponding chapter of the ECO (chapter B78, Sahovski Informator Beograd 2002). Also, the variation would have lost its popularity if it didn't please thousands of chess players, making them feel the opening is adapted to their personal tastes and styles (which, again, depends on its adaptive structures).

    Calvin's (1997) "six essentials" to qualify a process as Darwinian seem to be at work in the memetic evolution of chess openings, causing "quality" to emerge in many, but not all of the variations present in the chess hyper-memomic tree (an example of such quality is the one shown in figure 9).

    1. and 2. Copied patterns - Moves, positions, and entire variations are copied to the literature, and to future games, see sections 4.1 and 4.2 - of these, moves deserve a special attention, because they are the instructive replicators prescribing the phenotypic characteristics of games (section 1).

    3. Variant moves (novelties) are created by players; they may even be recreated, after disappearing from tournaments.

    4. Moves compete with their alleles, both as behavioural phemes, for a limited number of games in tournaments, and as memes in the minds of players (see sections 4 and 5).

    5. The competition between moves is biased by the environment (minds of players); proliferation of moves is potentiated by the effects they cause in games and players: good games, openings with even chances for both sides (section 7.1), satisfaction of player's tastes and styles.

    6. Changes in variations are little sidesteps from an already good (and usually already abundant) variation. Changes in openings and variations are not random jumps from some standard starting position (to paraphrase Calvin 1997).

    The process of evolution of openings recursively builds high quality structures following this algorithm. What happens is not a simple building of structures by selective elimination of some sub-structures (a "carving" selective process, Calvin 1997). No. Here is again the opening sequence that leads to the position of figure 9:
    1.e4 c5 2.Nf3 d6 3.d4 cd4 4.Nd4 Nf6 5.Nc3 g6 6.Be3 Bg7 7.f3 0-0 8.Qd2 Nc6 9.Bc4 Bd7 10.0-0-0 Rc8 11.Bb3 Ne5 12.h4 h5 13.Bg5 Rc5
    The shown moves, have been in allelic rivalry with other moves for decades, some for centuries. Earlier moves (say 1.e4, 2.Nf3 or 5...g6) have alleles that, like them, are very prolific, leading to similarly popular branches of the hyper-memomic tree of openings. Later in the variation (from the 9th move onwards), most moves displayed are far more prolific than their alleles (with the exception of 10...Rc8, which has 10...Qa5 as an equally successful allele). In other words, for the branches of the tree derived from the position set after the 8th move, only two main variations got high proliferation (the shown variation, encompassing 10...Rc8, and the variation encompassing its successful allele, 10...Qa5). These two variations (a) 9.Bc4 Bd7 10.0-0-0 Rc8 11.Bb3 Ne5 12.h4 h5 13.Bg5 Rc5, the one shown; and b) 9.Bc4 Bd7 10.0-0-0 Qa5 11.Bb3 Rfc8 12.Kb1 Ne5) got a proliferation and evolutionary success unmatched by the hundreds of alternative variations ever played in this branch (these hundreds of alternatives are described in chapters B78 and B79 of the ECO - Sahovski Informator Beograd 2002), let alone the millions of possible variations that don't appear in the ECO. Therefore, strong selection operated (4th and 5th essentials) between variations and their underlying moves. Moreover, the selective process was recursive (for example, moves 10...Rc8, 12.h4 and 13...Rc5 appeared as novelties at different times; each time they caused small improvements in already good structures (6th essential)).

    Therefore we can say that opening variations that get high proliferation (like the one shown above) come into existence through a selective process of which moves are the main selected entities. The process is truly Darwinian because of its recursiveness and gradualism: variations are improved through time step by step, in a gradualist fashion, each novelty move potentially adding a little to the quality of the variation. The evolutionary result is the emergence of adaptation (though not as fine-tuned as the adaptations of living organisms) in the tiny minority of variations that achieve high proliferation. Figure 10 illustrates the processes at work.

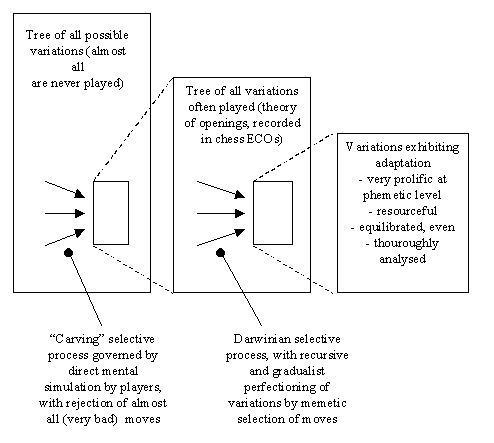
    Figure 10. Selective processes at work in chess. From the gigantic set of all possible moves and variations (leftmost rectangle), players select a smaller set of moves that "make sense" (that is, they are neither obvious losing moves nor irrational) and play them very often. This "carving" selective process results in the set of variations comprising the theory of openings (recorded in ECOs). Year after year, these ones are evolving, through a selective process truly recursive and gradualistic (and independent of the mental simulation performed by individual players in games), and the result is the improvement of a limited set of variations (rightmost rectangle) very prolific, adapted, equilibrated (even for both sides), and resourceful.

    7.3. Inter-specific arms races

    As pointed out in the last section, openings and variations evolve high quality structures to please players, producing playable, resourceful, and equilibrated games. There may be cases in which two different openings (species) are engaged in an arms race to overcome each other in this regard. This effect is particularly interesting when both openings are played often, and compete for the same ecological niche (psychology of players). For example, the King's Indian opening (defined by 1.d4 Nf6 2.c4 g6 3.Nc3 Bg7 4.e4 d6) and Indo-Benoni opening (defined by 1.d4 Nf6 2.c4 c5 3.d5 e6 4.Nc3 ed5 5.cd5 d6) are played very often, are both a choice of the player with Black, are both a response to the White move 1.d4, and both lead to sharp positions, so they may be engaged in an enduring arms race for resourcefulness, sharpness, equilibrium, etc, in order to not lose their "market share" among players whose style urges them to respond to 1.d4 with a sharp and resourceful defence.

    8. Conclusion

    This article aims to build just a very provisional starting point for the study of chess in a memetic perspective. I've put emphasis on memes associated with chess moves because moves are particulate instructive replicators that wholly prescribe the characteristics of other, more complex, chess entities: positions, games, and openings. Chess games are usefully conceptualised as the main interactors or organisms of chess moves (and section 6 draws parallels between them and living organisms). Moves made early in the opening are especially interesting because they replicate themselves more often to the literature and to other games, that is, openings and their branches (variations) are carriers of long-lasting move memes (section 3). Following on from Langrish (1999), it is interesting, in future models of chess meme transmission, to consider move memes divided into two fundamental categories: recipemes (just the knowledge of the move and its associated position) and selectemes (opinions about its worth). Selectemes about a move often change in the minds of players in the wake of outcomes of new masterly games containing the move, and upon new analyses of it appearing in chess informants, other periodicals and encyclopaedias. Selectemes about a move also often depend on a player's belief about whether the move fits in with his or her particular style of play. Future population memetical studies of chess should focus on populations of games (tournaments) recorded in periodicals, in move memes can be said to be expressed behaviourally as phemes.

    If we consider each opening position as an operational locus in which several possible moves compete in allelic rivalry, we get a useful memetical framework that helps us to understand the evolutionary processes of chess openings. In this framework, the opening of a given game is its memome, classified openings (defined by a set of opening moves) are species, its variations are sub-species, and the entire theory of openings can be called Chess Openings Hyper-Memome or hyper-memomic tree, a hyper-memome of which all opening memomes are sub-branches. The hyper-memomic tree is always evolving new branches, thanks to the novelties created by chess players. Its memomics does not mirror the genomics of biology in every detail, but it still illuminates evolutionary processes of chess openings. These evolutionary processes include arms races between the White side and the Black side. Variations tend to be forgotten if they lead to the victory or crushing advantage of one side over the other. Therefore, evolutionarily successful variations lead to equilibrated positions, with even chances for both sides or slight White advantage, and they also tend to be agreeable, resourceful, rich in tactical and strategic possibilities for both sides, and capable of occupying psychological niches in players' psychology. To achieve all this, successful opening variations rely on high quality structures in the formations of the pieces, which are erected through the full Darwinian process working over their underlying move memes.

    Appendix – Algebraic notation of chess moves

    In the algebraic notation, the most widely used and the one used throughout this article, a move is written as follows: first a letter that designates the type of piece moved (K-king, Q-queen, R-rook, B-bishop, N-knight, or no letter for a pawn), followed by the destination square of the piece. Moves are numbered in relation to the beginning of the game. Examples:
    1. e4      White’s first move, a pawn is moved to the e4 square

    17. Nc5+   White’s 17th move, a knight is moved to the c5 square, giving check (check is indicated by the "+" sign)

    10...Bg7  Black’s 10th move, a bishop is moved to the g7 square ("..." indicates this is Black’s move)

    Exceptions:
    7. 0-0         castling (a move involving the king and the king-side rook simultaneously)

    7. 0-0-0      castling (a move involving the king and the queen-side rook simultaneously)

    7. cd4         a pawn in column c captures a piece in the d4 square

    49. e8Q       White promoted a pawn to queen in the e8 square
     

    Glossary

    COHM - Chess Opening Hyper-Memome, or Hyper-Memomic Tree - the entire theory of openings as recorded in the ECOs, a tree-shaped hyper-memome comprising many thousands of loci, in which allelic moves compete.
    COMRM - Chess Opening Move-Related Meme, any meme containing information about a chess opening move, such as the idea of the move itself, evaluative opinions about it, etc.

    ECO - Encyclopaedias of Chess Openings - encyclopaedias that gather, and order hierarchically chess openings played by masters. They include comments and evaluations of resulting positions by leading masters. Their content is the fast-evolving theory of openings.

    Move - a transition between two chess positions, through the move of usually one piece from one square to another; a move has associated memes in players' minds: the knowledge of the move itself, evaluative opinions about it (meta-memes), and others. At behavioural level, played moves are usefully conceptualised as phemes.

    Novelty - a new move in the theory of openings. A novelty creates both a new move (allele) in the position (locus) it belongs to, and a new position (locus).

    Opening - the initial part of a game, when most pieces are developed to occupy active places. The opening is most relevant to memetics because its positions and moves proliferate in the world of chess tournaments.

    Position - a given situation of the chessboard, encompassing the positions of all the pieces on it, at a given time during a game.

    Variation - a branch in the opening tree.

    References

    Ball, J. A. (1984) Memes as Replicators, Ethology and Sociobiology 5:145-161

    Best, M. L. (1997) Models for Interacting Populations of Memes: Competition and Niche Behaviour.
    Journal of Memetics - Evolutionary Models of Information Transmission1 .
    http://cfpm.org/jom-emit/1997/vol1/best_ml.html

    Calvin, W. H. (1997) The Six Essentials? Minimal Requirements for the Darwinian Bootstrapping of Quality, Journal of Memetics - Evolutionary Models of Information Transmission 1.
    http://cfpm.org/jom-emit/1997/vol1/calvin_wh.html

    Capablanca, J. R. (1952) Ajedrez Fundamental, Madrid: Marsiega

    ChessBase GmbH (2001a) ChessBase Chess Informant (CD-Rom database), advertised in ChessBase website http://www.chessbase.com/

    ChessBase GmbH (2001b) ChessBase Opening Encyclopaedia 2002 (CD-Rom database), advertised in ChessBase website http://www.chessbase.com/

    Cosmides, L. & Tooby, J. (1981) Cytoplasmic Inheritance and Intragenomic Conflict, Journal of Theoretical Biology 89:83-129

    Dawkins, R. (1976) The Selfish Gene, Oxford: Oxford University Press

    Dawkins, R. (1978) Replicator Selection and the Extended Phenotype, Zeitschrift fur Tierpsychologie 47:61-76

    Dawkins, R. (1982) The Extended Phenotype, Oxford: Oxford University Press

    Dawkins, R. (1986) The Blind Watchmaker, New York: W. W. Norton

    Dawkins, R. (1995) River Out of Eden, New York: Basic Books

    Dawkins, R. & Krebs, J. R. (1979) Arms Races Between and Within Species, Procedures of the Royal Society of London B 205:489-511

    de Firmian, N. (1999) Modern Chess Openings, New York: Times Books

    Dennett, D. C. (1995) Darwin's Dangerous Idea, London: Penguin Books

    FIDE - Fedération Internationale des Échecs (2001) "Players and Rating" report, at FIDE website http://www.fide.com/

    Fine, R. (1956) The Psychology of the Chess Player, New York: National Psychological Association for Psychoanalysis

    Fine, R. (1990) The Ideas Behind Chess Openings, New York: Times Books

    Golombek, H. (1954) The Game of Chess, London: Penguin Books

    Hales, D. (1998) An Open Mind is not an Empty Mind: Experiments in the Meta-Noosphere, Journal of Artificial Societies and Social Simulation,vol1, no 4, http://www.soc.surrey.ac.uk/JASSS/1/4/2.html

    Horowitz, A. (1961) The Golden Treasury of Chess, New York: Barnes and Noble

    Hull, D. L. (1988) Science as a Process: An Evolutionary Account of the Social and Conceptual Development of Science, Chicago IL: University of Chicago Press

    Hurst, L. D. & Atlan, A. & Bengtsson, B. O. (1996) Genetic Conflicts, Quarterly Review of Biology 71:317-364

    Interchess BV (2001) New in Chess CD-Rom Series (CD-Rom database), advertised in New in Chess website, http://www.newinchess.com/

    Jan, S. (2000). Replicating Sonorities: Towards a Memetics of Music .
    Journal of Memetics - Evolutionary Models of Information Transmission 4, http://cfpm.org/jom-emit/2000/vol4/jan_s.html

    Karpov, A. (1988) The Semi-Open Game in Action, London: B. T. Batsford

    Keene, R. (1990) Chess: an Illustrated History, New York: Phaidon Press

    Keverne, E. & Martel, F. & Nevison, C. (1996) Primate Brain Evolution: Genetic and Functional Considerations, Procedures of the Royal Society of London, 262:689-696

    Kotov, A. (1982) Entrene como un Gran Maestro, Madrid: Editorial Ricardo Aguilera

    Langrish, J. Z. (1999). Different Types of Memes: Recipemes, Selectemes and Explanemes,
    Journal of Memetics - Evolutionary Models of Information Transmission 3 ,  http://cfpm.org/jom-emit/1999/vol3/langrish_jz.html

    Lynch, A. (1998) Units, Events and Dynamics in Memetic Evolution.
    Journal of Memetics - Evolutionary Models of Information Transmission2,
    http://cfpm.org/jom-emit/1998/vol2/lynch_a.html

    Maynard Smith, J. (1998) Evolutionary Genetics, 2nd edition, Oxford: Oxford University Press

    Moore, T. & Haig, D. (1991) Genomic Imprinting in Mammalian Development: a Parental Tug-of-War, Trends in Genetics 7:45-49

    Pocklington, R. & Best, M. L. (1997) Cultural Evolution and Units of Selection in Replicating Text, Journal of Theoretical Biology 188:79-87

    Sahovski Informator Beograd (1978) Encyclopaedia of Chess Openings, volume B (1978 edition) , Belgrad: Sahovski Informator Beograd

    Sahovski Informator Beograd (1998) Chess Informant, Belgrad: Sahovski Informator Beograd

    Sahovski Informator Beograd (2002) Encyclopaedia of Chess Openings, volume B (2002 edition) , Belgrad: Sahovski Informator Beograd

    Van Valen, L. (1973) A New Evolutionary Law, Evolutionary Theory 1:1-30

    Wilkins, J. S. (1998) What's in a Meme? Reflections from the perspective of the history and philosophy of evolutionary biology. Journal of Memetics - Evolutionary Models of Information Transmission 2 , http://cfpm.org/jom-emit/1998/vol2/wilkins_js.html

    © JoM-EMIT 2002

    Back to Issue 2 Volume 6